KNOW MORE
Laureate LanguagesYMLP can provide you with resources for designing a project, creating a call for laureate proposals, advertising for laureate nominations, identifying a laureate, and more!
did you know?
Why are YMLP’s Mathematician Laureates called artists?
The background for this approach is influenced by the philosophers Nelson Goodman and Hannah Arendt.
Goodman’s book, Languages of Art: An Approach to a Theory of Symbols, deals with aesthetics, which is a branch of philosophy that tries to understand creativity, the nature of beauty and taste, and the ways that art can instigate thinking, feeling, and action in others. What sort of criteria can be used to distinguish laureates, judge the strength of a proposal, or feel like a project is making a genuine impact? Our guidance for these areas of YMLP emphasize mathematics as ‘bigger than’ school math, and deeper in its usefulness as a cultural resource, and youth as genuine ‘leaders’ as described by Roger Hart in his UNICEF Report, Children’s Participation: From Tokenism to Citizenship.
Nelson Goodman
Goodman’s Influence on YMLP
Those familiar with the work of Nelson Goodman will recognize that the five attributes listed here are variations on his properties (or ‘symptoms’) of when art is taking place. Rather than trying to decide whether we should call something “art” or not, Goodman helps us to recognize when art is “happening.” If you see a young person helping a group of people to do some or all of these things, they are acting together as artists:
Syntactic Density
The finest of differences (in the symbols employed) constitute a difference (among the symbols).
Semantic Density
There are symbols to refer to the finest differences in the world to which the symbols refer.
Relative Repleteness
Comparatively many aspects of the symbols are significant.
Exemplification
When a symbol serves as a sample of properties it literally or metaphorically possesses.
Multiple & Complex Reference
Where a symbol performs several integrated and interactive referential functions (some direct and some mediated through other symbols).
The shift, for Goodman, is from the question, “What is art?”, to “When is art?” He accomplishes this through a shift from thinking of art as a kind of language or tool of communication, to a kind of system of symbols. This changes the world of looking at and making art:
Language of Art
- Symbol Systems
- Resemblance
- Representation
- Symbol Systems
- Resemblance
- Representation
- Denotation
- Exemplification
- Authenticity: autographic
- Authenticity: allographic
- Notation
- Score, sketch, script, etc.
- Denotation
- Exemplification
- Authenticity: autographic
- Authenticity: allographic
- Notation
- Score, sketch, script, etc.
Mathematical Arts
Sometimes, Mathematician Laureates enact mathematics as dance
The dancer/choreographer mathematician is primarily concerned with:
Body
Body
Their materials are their own and others’ bodies; they isolate body parts in order to accomplish their goals.
Actions
Actions
They do things with and through their bodies.
Time
Time
The work has a beginning and end, rhythm, pulse, may or may not be free-flowing or determined, but nevertheless uses time as an element.
Space
Space
Their actions are in relation to the place in which they find or put their bodies, and their work involves interaction with this place.
Energy
Energy
Are they forceful, graceful, heavy, displaying bodies? Do things occur in time suddenly or smoothly? Delicately?
Mathematical Arts
Sometimes Mathematicians Laureates enact mathematics as sculpture
The sculptor mathematician is primarily concerned with:

- Line
- Plane
- Color
- Value
- Form
- Space – both positive and negative
- Subject
- Line
- Plane
- Color
- Value
- Form
- Space – both positive and negative
- Subject
- Theme
- Balance – symmetrical or asymmetrical
- Proportion or altered proportion
- Emphasis
- Rhythm and Repetition
- Unity and Variety
- Environmental
- Installation
- Additive or Subtractive
- Assemblage
- Casting
- Representational, abstract or non-objective
- Theme
- Balance – symmetrical or asymmetrical
- Proportion or altered proportion
- Emphasis
- Rhythm and Repetition
- Unity and Variety
- Environmental
- Installation
- Additive or Subtractive
- Assemblage
- Casting
- Representational, abstract or non-objective
Hannah Arendt
Hannah Arendt & YMLP
Through the act of transporting, translating the into fiction, artists are able to keep alive concepts, thoughts, and ideas that have lost their presence in the world. Concepts and ideas that have become dubious can be re-examined through art. (Reference – Knott, 2013; p. 72)
The philosopher Hannah Arendt believed that the arts (and for us, mathematical practices are art!) hold particular promise for restoring connections to the world that have been severed by totalitarianism.
In particular, Arendt expressed four ways in which the (mathematical) arts can help us to unlearn some commonly held beliefs and notions of realilty:
- Laughter
- Translation
- Forgiveness
- Dramatization
And in this respect, it is common to find mathematician laureates using mathematics in one of these four ways. Their mathematical practices disrupt assumptions and perceptions, and help their communities to challenge them.
Laughter
Is one of Hannah Arendt’s most powerful strategies for developing the intellectual freedom that permits her to unlearn dominant philosophical and cultural prejudices. Perhaps YMLP laureates use to some extent a reframing of common-sense assumptions that makes everyday life look or feel absurd. Innocent surprise at how things keep working as they do can open up new possibilities for action.
Translation
From one language to another, or from one perspective to another, might be a technique leading to the reframing that causes laughter as a form of unlearning our routines of life. Or, it might be a way of exploring new forms of knowing. An important research-based recommendation for mathematics teaching and learning is to have students ‘translate’ from one representation or picture of a situation to another, and to then look for the commonalities that are exposed through the different ways of looking at things. Also, just as Hannah Arendt would write in English, French or Spanish, rather than her mother tongue of German, in order to force herself to be very careful about word choices and meaning, YMLP project use mathematics rather than more obvious ways of communicating about situations in the world, for similar purposes of clarity and interpretation.
Forgiveness
Has a particular meaning for Hannah Arendt which we can use to unlearn anger and disappointment with problems that we see in the world. It involves accepting things that have happened and not wallowing in persistent re-telling of the stories of unfairness. Instead, what we do is begin with the reality that the problems exist in the world, and we now will refuse to let those responsible and our anger toward them to influence how we proceed. We more ahead without worrying all that much about previous grievances, without forgetting them.
Dramatization
For YMLP as for Hannah Arendt, recognizes the power of narrative and storytelling in instigating action in the world. One the one hand, mathematical stories about community issues reveal new insights because of their ability to conjure laughter, translation and forgiveness. On the other hand, stories are told form particular perspectives on the situation, so that we need to include the stories of many different constituencies, and from diverse perspectives.
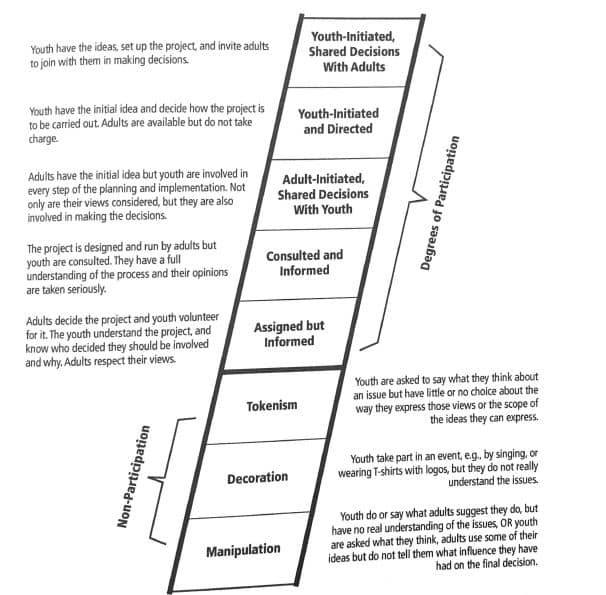
Roger Hart & The Ladder of Participation
A significant feature of YMLP is how it values the input and leadership of youth, rather than facilitating their participation in adult-designed activities.
Instead of teaching children, YMLP facilitates community organizations in their support of youth leaders. Roger Hart’s report to UNICEF in the 1970s, Children’s Participation, uses a ladder of possibilities for how we can understand the inclusion of youth in community projects. YMLP projects are at or near the top of Hart’s ladder:
YMLP projects are at their best when they are youth-initiated, with adults as shared decision-makers. Youth are the directors of their own projects. Adults rarely if ever do more than indicate their interest in types of projects or current issues that their organization wants to address. If a project idea begins with youth as consultants or as trainee workers, then it should be re-thought and re-designed by a YMLP laureate themself in order to meet YMLP goals.